Mechanics: State, explain, analyse and apply principles of Newton’s first and second laws of motion
Unit 1: Newton’s first law of motion
Leigh Kleynhans
Unit outcomes
By the end of this unit you will be able to:
- Identify whether forces applied on a mass are balanced or not; and determine the net force.
- Define inertia and give examples.
- Define Newton’s first law of motion.
- Relate Newton’s first law of motion to examples.
What you should know
Before you start this unit, make sure you can:
- Draw vector diagrams for two forces acting on an object and determine the resultant. Revise subject outcome 2.2 unit 1 to help you with this.
- Determine the components of a force. Revise subject outcome 2.2 unit 2 to help you with this.
- Draw labelled free-body diagrams of objects in various situations. Revise subject outcome 2.2 unit 3 to help you with this.
- Draw free-body diagrams of parallel forces. Revise subject outcome 2.3 unit 2 to help you with this.
- Draw free-body diagrams of non-parallel forces. Revise subject outcome 2.3 unit 2 to help you with this.
Introduction
Parts of the text in this unit were sourced from Siyavula Physical Science Gr 11 Learner’s Book, Chapter 21, released under a CC-BY licence
In this unit you will learn how force affects motion. This is a universal principle that is described by Newton’s first law of motion. Newton’s first law of motion helps us to understand what makes an object stay at rest or keep it moving uniformly. Once you understand this relationship between force and motion, you will be able to identify examples of how Newton’s first law applies in the world around us.
Finding the net or resultant force
You have already learnt how to determine the resultant or net force acting on an object, [link to Phys Sci L3 SO 2.2 Unit 1] and to draw free-body diagrams.

In figure 1, the is obtained by adding the two vectors, taking their directions into account.
For the example, assume that the positive direction is to the right, then:
[latex]\scriptsize \begin{align*}{{F}_{{net}}}&={{F}_{1}}+{{F}_{2}}=-6+(+4)=-2\\{{F}_{{net}}}&=2\text{ N to the left}\end{align*}[/latex]
Remember that a negative answer means that the force acts in the opposite direction to the one that you chose to be positive. You can choose the positive direction to be any way you want, but once you have chosen it, you must use it consistently for that problem. These forces are referred to as being . They act in the same plane but are different magnitudes, therefore there will be a resultant force in the direction of the larger force.
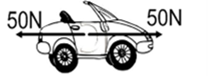
In figure 2, the forces acting in the same plane are equal in magnitude. When you do the vector addition to find the resultant, you will get [latex]\scriptsize 0\text{ N}[/latex] as the answer. This simply means that the forces are , and the resultant is zero.
The principle of vector addition can be used to find the net or resultant force in the horizontal or vertical planes, or on inclined planes.
Exercise 1.1
For the following situations, say whether the forces are balanced or unbalanced and determine the net force:
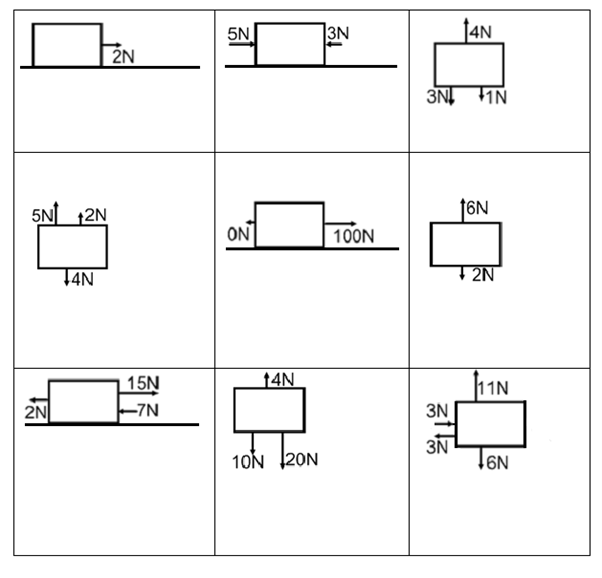
The full solution is at the end of the unit.
Inertia
The property of an object to continue in its current state of motion unless acted upon by a net force, is called . All objects with mass will have inertia. If an object is stationary, it will not move unless a net force acts on it, and if an object is moving at constant velocity, it will not speed up, slow down, change direction or stop unless a net force acts on it.
Let us consider the following situations:
- An ice skater pushes herself away from the side of the ice rink and skates across the ice. She will continue to move in a straight line across the ice unless something stops her.
- If we kick a soccer ball across a soccer field, it continues to move in the direction in which we kicked it, unless another force acts on it to stop it or change its direction.
In real life there is always friction between surfaces or even friction between moving objects and the air, so we never experience the situation of the objects continuing to move forever. Friction is what causes moving objects to slow down and eventually stop. Without friction, the inertia of an object will result in it continuing to move at a constant velocity forever! So, if we were able to remove friction from the above situations, the ice skater and the soccer ball will continue moving at constant velocity forever.
Newton’s first law of motion
Sir Isaac Newton was a scientist who lived in England (1642–1727) who was interested in the motion of objects under various conditions. He suggested that a stationary object will remain stationary unless a force acts on it and that a moving object will continue moving unless a force slows it down, speeds it up or changes its direction of motion. From this he formulated what is known as .
Newton’s first law of motion
An object continues in a state of rest or uniform motion (motion with a constant velocity) unless it is acted on by an unbalanced (net or resultant) force.
Let us look at the following examples which illustrate this law.
Seat belts
We wear seat belts in cars for safety. This is to protect us when the car is involved in an accident. If a car is travelling at [latex]\scriptsize 120\text{ km}\text{.}{{\text{h}}^{{-1}}}[/latex], the driver and passengers in the car are also travelling at [latex]\scriptsize 120\text{ km}\text{.}{{\text{h}}^{{-1}}}[/latex]. When the car suddenly stops a force is exerted on the car (making it slow down), but if the driver and passengers are not wearing seatbelts, no force is exerted on them. The driver and passengers will carry on moving forward at [latex]\scriptsize 120\text{ km}\text{.}{{\text{h}}^{{-1}}}[/latex] according to Newton’s first law. If they are wearing seat belts, the seat belts will stop them by exerting a force on them and thus prevent them from getting hurt.

Rockets and Spaceships
When a spaceship is launched into space, the force of the exploding gases pushes the spaceship through the air into space. Once it is in space, the engines are switched off and it will keep on moving at a constant velocity. There is no air friction or significant gravitational force to slow it down. If the astronauts want to change the direction of the spaceship, they need to fire an engine. This will then apply a force on the spaceship and it will change its direction.

Activity 1.1: Investigate balanced and unbalanced forces and demonstrate Newton’s first law of motion
Time required: 20 minutes
What you need:
- an internet connection
What to do:
- Use this link to access a computer simulation on how Newton’s first law of motion relates to the movement of an object.
- Click on the first of the four windows, labelled Net Force. You will see a cart with a rope attached to either side of it, and blue and red figures underneath.
- Move the smallest blue figure up to the rope (see figure 5), and notice that there is now an applied force to the left. Click on the button labelled Go! and see what happens as a result of this force.
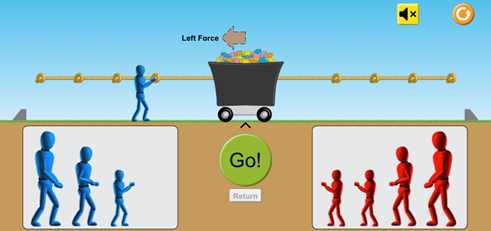
Figure 5: An unbalanced force pulling towards the left - To start everything again click the reset button (orange button with white circular arrow ↺)
- Move the smallest blue figure up to the rope again, and this time add the smallest red figure to the other side of the rope (see figure 6).
- What do you notice about the forces on the cart?
- What happens when you press the Go! button?
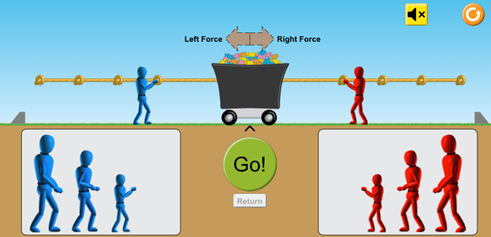
Figure 6: Balanced forces
- Now add another of the smallest blue figures to the rope, and see what happens to the forces.
What is the direction of the net force? (You can see the net force by selecting the square labelled Sum of Forces, as shown in figure 7).
Figure 7: Select the Sum of Forces option - Press the Go! Button. Once the cart is moving, add another smallest red figure to the other side of the rope so that there is no net force.
- What happens to the movement of the cart when there is no net force on it while it is moving?
- What do you need to do to stop the cart from moving? (Experiment with adding figures to the rope to see how to stop the cart.)
- Use your observations to complete these sentences:
- When the cart is standing still, and the left and right forces on the cart are balanced, the cart will _____________________________.
- When the cart is moving to the left, and the left and right forces on the cart are balanced, the cart will _____________________________.
- What you need in order to change the movement of the cart is ______________________.
What did you find?
In this simulation you would have observed the following:
- When one of the blue figures was pulling on the left rope, there was only one applied force to the left, which meant that there was a net (unbalanced) force on the cart. When the button labelled Go! was clicked, the cart started accelerating towards the left as a result of this net force.
- .
- When there was a blue figure and a red figure pulling on opposite sides of the cart, the force to the left was balanced by the force to the right, and as a result there was no net force on the cart.
- When you pressed the Go! button there was no movement, since there was no net force to cause a change in the cart’s state of motion.
- When another small blue figure was added to the left rope, the forces were no longer balanced. There was a net force acting on the cart to the left. This caused the cart to accelerate to the left.
- .
- Once the cart was moving, when one of the smallest red figures was added to the right side of the cart, the forces were balanced and there was no net force. As a result the cart kept moving to the left with constant speed. (You can check this by clicking on the box labelled Speed at the top right of the screen).
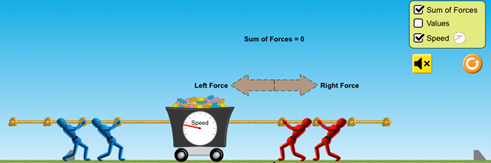
Figure 8: Balanced forces result in constant velocity - What you need to do to stop the cart from moving is to add another red figure so that there is a net force to the right. This net force will cause the cart to slow down until it stops. If you don’t pause the motion once it has stopped the net force to the right will then cause the cart to accelerate towards the right.
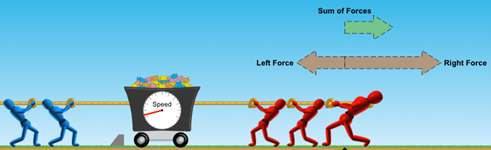
Figure 9: A net force to the right will cause a cart moving to the left to slow down
- Once the cart was moving, when one of the smallest red figures was added to the right side of the cart, the forces were balanced and there was no net force. As a result the cart kept moving to the left with constant speed. (You can check this by clicking on the box labelled Speed at the top right of the screen).
- The completed sentences are shown below:
- When the cart is standing still, and the left and right forces on the cart are balanced, the cart will remain standing still.
- When the cart is moving to the left, and the left and right forces on the cart are balanced, the cart will continue moving to the left with a constant speed.
- What you need in order to change the movement of the cart is a net (or unbalanced) force.
These sentences are a description of Newton’s first law. (Have a look at it again, and compare it with the observations you made during the simulations.)
Example 1.1
Why do passengers get thrown to the side when the car they are driving in goes around a corner?
Solution
Step 1: What is happening before the car turns?
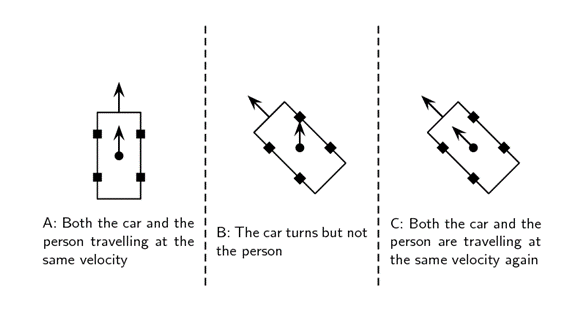
Before the car starts turning both the passengers and the car are travelling at the same velocity (see picture A in figure 10).
Step 2: What happens while the car turns?
The driver turns the wheels of the car, which then exerts a force on the car and the car turns. This force acts on the car but not the passengers, hence (by Newton’s first law) the passengers continue moving with the same original velocity (see picture B in figure 5).
Step 3: What happens to the passengers?
If the passengers are wearing seat belts, they will exert a force on the passengers until the passengers’ velocity is the same as that of the car (see picture C in figure 5). Without a seat belt the passengers will continue to move in their original direction (forward) and hit the side of the car.
Exercise 1.2
Explain how the following real-life examples illustrate Newton’s first law of motion.
- Coin drop

.
The piece of card is flicked horizontally by the finger and the coin falls into the glass. - Pile of blocks

.
Stack a pile of wooden blocks on a table. Hit the lower block hard and fast without touching the others. The bottom block moves away quickly, the other blocks drop vertically onto the table but do not move horizontally like the bottom block. - Car crash
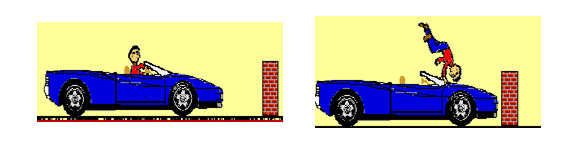
.
A person is in a car and is not wearing a seatbelt. The car crashes into a wall and the driver is ‘thrown’ from the car.
The full solutions are at the end of the unit.
Note
Watch this video to see a collection of inertia demonstrations.
Summary
In this unit you have learnt the following:
- When two or more forces in one plane are equal in magnitude but act in opposite directions they are referred to as balanced forces.
- When two or more forces acting in one plane and in opposite directions are not equal they are referred to as unbalanced forces.
- When forces are unbalanced, there will be a net or resultant force on the object.
- Net or resultant forces can be calculated using vector addition: [latex]\scriptsize {{F}_{{net}}}={{F}_{1}}+{{F}_{2}}[/latex] (remember to choose a direction as positive).
- Inertia is the property of an object that causes it to resist a change in its state of rest or uniform motion.
- Newton’s first law of motion states that an object continues in a state of rest or uniform motion (motion with a constant velocity) unless it is acted on by an unbalanced (net or resultant) force.
Unit 1: Assessment
Suggested time to complete: 20 minutes
- State whether the following situations involve balanced or unbalanced forces on the underlined object. If the forces are unbalanced, calculate the net force:
- a book rests on a table
- a man pushes a wheelbarrow with a force of [latex]\scriptsize 120\text{ N}[/latex] and the frictional force of the surface is [latex]\scriptsize 70\text{ N}[/latex]
- a skydiver has a weight of [latex]\scriptsize 720\text{ N}[/latex] and the air resistance is [latex]\scriptsize 600\text{ N}[/latex]
- a briefcase is held by a man.
- Explain the term inertia.
- State Newton’s first law of motion.
- Thabo has attached a rope to a box, and is pulling on the rope, but the box does not move at all.
- What is the net force on the box?
- Explain what is keeping the box from moving.
- A mother is sitting in the front passenger seat of a car with a child on her lap, and is not wearing a seat belt. Explain why this is dangerous, especially for the child.
- Use Newton’s first law of motion to explain why it is dangerous to stand in a moving bus.
- A girl on a train jumps up while a train is moving forward with a constant velocity. What will her position be when she lands, relative to her position when she took off upwards. Explain your answer referring to Newton’s first law of motion.
The full solutions can be found at the end of the unit.
Unit 1: Solutions
Exercise 1.1
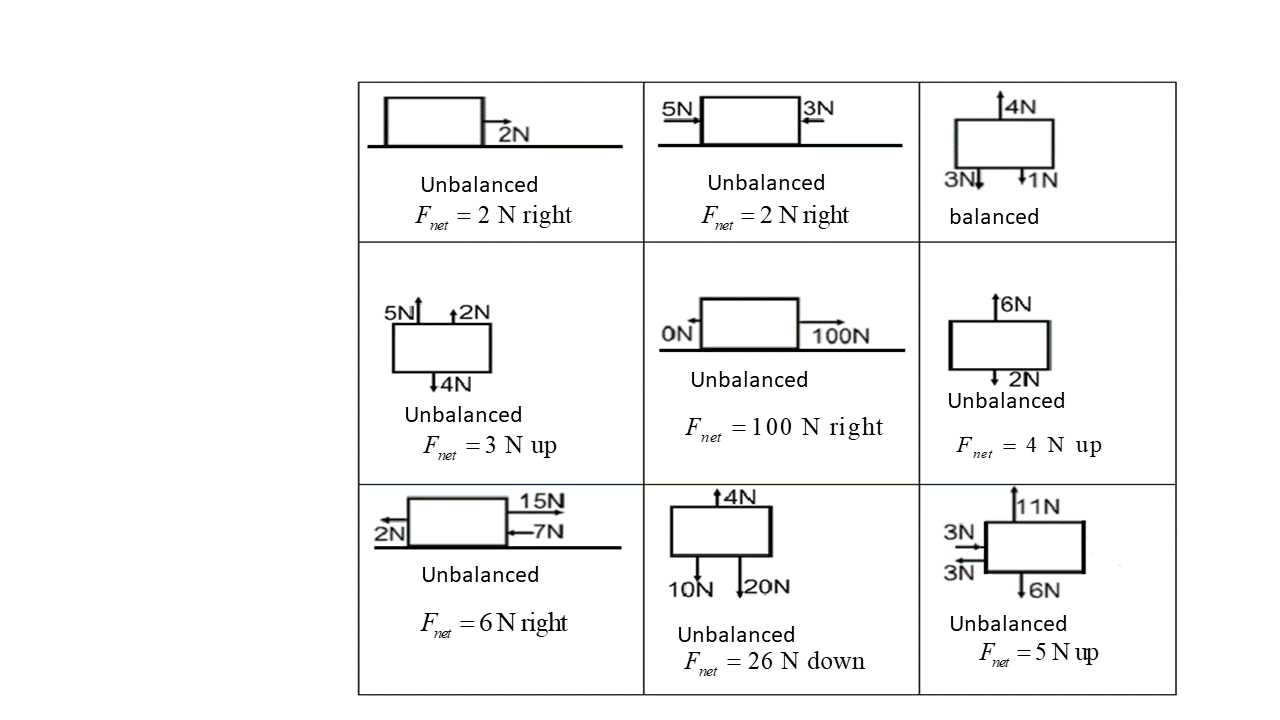
Exercise 1.2
- An external force is applied to the piece of card and not to the coin. The inertia of the coin causes it to resist a change in its state of motion and it remains in its original position. (The friction of the card on the coin is not enough to move it sideways.) Without the card in place the coin falls into the glass. (If the card is moved slowly and not flicked, friction will act on the coin and it will move sideways with the card.)
- An external force acts on the bottom block so it moves sideways. The inertia of the upper blocks causes them to resist a change in their state of motion and they remain in their original horizontal position. (As in question 1, if the lower block is moved slowly, the frictional force between the blocks will act on the upper blocks and move them sideways.)
- When the car hits the wall, the wall applies an external force to the car and it stops. The inertia of the driver causes him to resist a change in his state of motion and he continues to move forward at his original velocity. Therefore, he lands up on the front bonnet of the car.
Unit 1: Assessment
- .
- balanced forces (the book is not moving)
- unbalanced
[latex]\scriptsize \begin{align*}{{F}_{{net}}}&={{F}_{1}}+{{F}_{2}}=120+(-70)=50\\&=\text{50 N in the original direction}\end{align*}[/latex] - unbalanced
[latex]\scriptsize \begin{align*}{{F}_{{net}}}&={{F}_{1}}+{{F}_{2}}=720+(-600)=120\\&=\text{120 N down}\end{align*}[/latex] - balanced
- Inertia is the property that objects have that causes them to resist a change in their state of motion.
- An object continues in a state of rest or uniform motion (motion with a constant velocity) unless it is acted on by an unbalanced (net or resultant) force.
- .
- net force is zero (object is not moving)
- there must be a frictional force that balances the applied force (same size but acting in the opposite direction)
- The mother and child have inertia. They will therefore, according to Newton’s first law of motion, resist a change in their state of motion. During a collision, the car will slow down because a resultant force acts on it. No resultant force acts on the mother and child, so both will continue moving forward at their original velocity. They will therefore hit the dashboard or windscreen and suffer severe injury. This is particularly dangerous for the child as when a resultant force (the dashboard) stops the child, the mother will still continue at her initial velocity and squash the child.
- If the bus comes to a sudden stop, a person who is standing will resist a change in their state of motion because of inertia. They will obey Newton’s first law of motion. Their feet will stop with the bus as an unbalanced force (friction) acts on them, but their body will continue to move at the original velocity and this will cause them to fall over.
- The girl has inertia, and will obey Newton’s first law of motion. She will continue in her original state of forward motion even when she is not touching the floor of the train. No unbalanced forces act on her in the horizontal plane. So, she will land in her original position on the floor of the train.
Media Attributions
- img01_Figure1 © Siyavula is licensed under a CC BY-NC-ND (Attribution NonCommercial NoDerivatives) license
- img02_Figure2 © Siyavula is licensed under a CC BY-NC-ND (Attribution NonCommercial NoDerivatives) license
- img03_Ex1.1 © DHET is licensed under a CC BY (Attribution) license
- img04_Figure3 © Siyavula is licensed under a CC BY-NC-ND (Attribution NonCommercial NoDerivatives) license
- img05_Figure4 © Pixabay is licensed under a CC0 (Creative Commons Zero) license
- img06_Figure5 © PHeT Simulations is licensed under a CC BY (Attribution) license
- img07_Figure6 © PHeT Simulations is licensed under a CC BY (Attribution) license
- img08_Figure7 © PHeT Simulations is licensed under a CC BY (Attribution) license
- img09_Figure8 © PHeT Simulations is licensed under a CC BY (Attribution) license
- img10_Figure9 © PHeT Simulations is licensed under a CC BY (Attribution) license
- img11_Figure10 © Siyavula is licensed under a CC BY-NC-ND (Attribution NonCommercial NoDerivatives) license
- img12_Ex1.2Q1 © L Kleynhans is licensed under a CC BY (Attribution) license
- img13_Ex1.2Q2 © L Kleynhans is licensed under a CC BY (Attribution) license
- img14_Ex1,2Q3 © L Kleynhans is licensed under a CC BY (Attribution) license
- img15_Ex1.1answer © L Kleynhans is licensed under a CC BY (Attribution) license
the overall effect of all the forces acting on an object
when the forces in one plane acting in opposite directions are unequal in magnitude and therefore there is a resultant force in the direction of the larger force
when the forces in one plane acting in opposite directions are equal in magnitude and there is thus no resultant or net force in that plane
the property of an object that causes it to resist a change in its state of rest or uniform motion
an object continues in a state of rest or uniform motion (motion with a constant velocity) unless it is acted on by an unbalanced (net or resultant) force
