Unit outcomes

By the end of this unit you will be able to:

• Distinguish between concurrent and non-concurrent forces
• State and apply the two conditions for equilibrium with parallel forces.

What you should know

Before you start this unit, make sure you can:

• Define a force. Refer to level 2 subject outcome 2.2 unit 1 if you need help with this.
• Draw a force diagram. Refer to level 2 subject outcome 2.2 unit 2 if you need help with this.

Introduction

In this unit you will extend your knowledge of working with forces to situations where forces act on different points in an object. These are called non-concurrent forces. In this unit you will apply the concept of torque in solving problems that involve non-concurrent forces.

Non-concurrent forces

When forces are applied to a single point in an object at the same time, we say they act concurrently. Applied forces that act on different points in an object at the same time are said to act non-concurrently, as shown in figure 1. In the figure, [latex]\scriptsize {{F}_{1}}[/latex] and [latex]\scriptsize {{F}_{2}}[/latex] are parallel non-concurrent forces. [latex]\scriptsize {{F}_{3}}[/latex] and [latex]\scriptsize {{F}_{4}}[/latex] are non-parallel non-concurrent forces. The point P represents the pivot.

As you already know from your study of concurrent forces, an object will move in the direction of a non-zero resultant force. This also applies when forces act non-concurrently. However, because the forces do not act on the same point, the movement will not necessarily be in a straight line. Unbalanced non-concurrent forces applied to an object fixed at a point will cause the object to turn – or rotate – about that point (see figure 1).

Activity 1.1: Observe the effect of non-concurrent forces

Time required: 5 minutes

What you need:

• a dowel stick of about 1 m
• a short cylinder (e.g. a toilet roll inner)
• a few coins, all of the same type
• sticky putty or modelling clay

What to do:

1. Place the cylinder on a table and stick it down with some sticky putty or modelling clay.
2. Place the dowel stick on the cylinder so that it is balanced. Where does the cylinder have to be, relative to the length of the dowel stick, to keep it balanced?
   
3. Now move the dowel stick more to one side. What do you notice?
4. Return the dowel stick back to the position where it is balanced. Place a coin on each side of the dowel stick.
   1. Where do the coins have to be placed to keep the system balanced?
   2. What happens when the coins are not placed at equal distances from the cylinder?
5. Return the dowel stick back to the position where it is balanced. Now place two coins on one side of the dowel stick, and one coin on the other side of the dowel stick. The coins should be placed at equal distances. What do you notice?

What did you find?

• Step 2: To balance the dowel stick, the cylinder must be in the middle of the stick. In other words, the distance from the cylinder to the tip of the dowel stick on each side must be the same.
• Step 3: If you move the dowel stick more to one side, the longer section dips downwards. The cylinder acts as the pivot.
• Step 4: If you place a coin (of the same type) at equal distances on each side of the pivot, the stick remains balanced.
  If the coins are placed at unequal distances, the system is unbalanced and the stick will dip downwards on the side where the coin is further from the pivot.
  
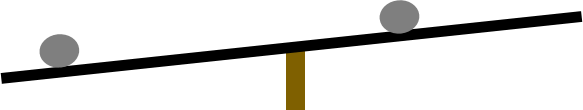
• Step 5: When the two coins are placed on one side of the stick, but only one coin on the other side, the stick dips downwards on the side of the two coins. This is because the downward force – in other words, the weight – is unbalanced, and greater on one side of the pivot.
  

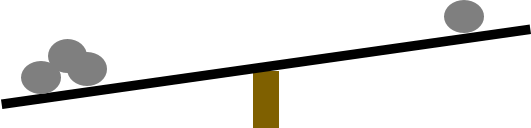
In activity 1.1, you observed rotation about a pivot. The rotation is caused by an unbalanced force. This quantity is called torque, which is a measure of the ability of a force to cause a rotation. Torque is usually represented by the symbol [latex]\scriptsize \tau[/latex] (the Greek letter ‘tau’) and calculated as the product of the force and its perpendicular distance from the pivot: [latex]\scriptsize \tau =F{{r}_{\bot }}[/latex]. The unit of torque is newton metres ([latex]\scriptsize \displaystyle \text{N}\text{.m}[/latex]).

Take note!

The torque of a force is also called its moment.

Conditions for equilibrium

You already know that to keep a system of forces in equilibrium, the resultant force must be zero. In other words, all the forces in the vertical direction must be balanced, as must all the forces in the horizontal direction, So, [latex]\scriptsize \displaystyle \Sigma {{F}_{x}}=0[/latex] and [latex]\scriptsize \displaystyle \Sigma {{F}_{y}}=0[/latex]. This is the first condition for equilibrium.

The second condition for equilibrium is that the sum of the clockwise torques must be equal to the sum of the anticlockwise torques: [latex]\scriptsize \displaystyle \Sigma {{\tau }_{{\text{clockwise}}}}=\Sigma {{\tau }_{{\text{anticlockwise}}}}[/latex].

Understanding what the conditions for equilibrium are allows us to solve problems in which a system of non-concurrent forces must be balanced.

Example 1.1

Example taken from Chapter 9 in OpenStax Physics, p. 322 released under a CC-BY licence

The two children shown in the diagram are balanced on a seesaw of negligible mass. The first child has a mass of [latex]\scriptsize 26.0\ \text{kg}[/latex] and sits [latex]\scriptsize 1.60\text{ m}[/latex] from the pivot.

1. If the second child has a mass of [latex]\scriptsize 32.0\ \text{kg}[/latex], how far is she from the pivot?
2. What is [latex]\scriptsize \displaystyle {{F}_{\text{p}}}[/latex], the force exerted on the seesaw by the pivot?

Solutions

1. We are asked for a distance. Thus, the second condition for equilibrium (regarding torques) must be used. Recall that the downward force exerted by each child – the weight – is calculated as [latex]\scriptsize W=mg[/latex].
   Thus: [latex]\scriptsize \displaystyle {{W}_{1}}={{m}_{1}}g=26.0\ \times \ 9.8=254.8\ \text{N}[/latex] and [latex]\scriptsize \displaystyle {{W}_{2}}={{m}_{2}}g=32.0\ \times \ 9.8=313.6\ \text{N}[/latex].
   [latex]\scriptsize \displaystyle \begin{align*}\Sigma {{\tau }_{{\text{clockwise}}}}&=\Sigma {{\tau }_{{\text{anticlockwise}}}}\\(254.8)(1.6)&=(313.6){{r}_{2}}\\\therefore {{r}_{2}}&=1.3\text{ m}\end{align*}[/latex]
   The second child has to sit [latex]\scriptsize \text{1}\text{.3 m}[/latex] from the pivot.
2. We know that the sum of the forces in the vertical direction is zero. Thus:
   [latex]\scriptsize \displaystyle \begin{align*}\Sigma {{F}_{y}}&=0\\{{F}_{\text{p}}}-254.8-313.6&=0\\\therefore {{F}_{\text{p}}}&=568.4\text{ N}\end{align*}[/latex]

Exercise 1.1

1. When opening a door, you push on it perpendicularly with a force of [latex]\scriptsize 55.0\text{ N}[/latex] at a distance of [latex]\scriptsize 0.850\text{ m}[/latex] from the hinges. What torque are you exerting relative to the hinges?
2. When tightening a bolt, you push perpendicularly on a wrench with a force of [latex]\scriptsize 165\text{ N}[/latex] at a distance of [latex]\scriptsize 0.140\text{ m}[/latex] from the centre of the bolt. How much torque are you exerting in newton metres (relative to the centre of the bolt)?
3. A beam of [latex]\scriptsize 2.4\ \text{m}[/latex] is balanced on a pivot. An object of [latex]\scriptsize 27\ \text{kg}[/latex] hangs from one end of the beam, [latex]\scriptsize 1.2\ \text{m}[/latex] from the pivot. Another object hangs on the other side of the pivot, [latex]\scriptsize 800\ \text{cm}[/latex] from the pivot. What is the mass of the second object if the beam is perfectly balanced?
   

The full solutions are at the end of the unit.

Summary

In this unit you have learnt the following:

• Non-concurrent forces are forces that act on different points of an object.
• Unbalanced non-concurrent forces applied to an object fixed at a point will cause the object to rotate about that point.
• Torque ([latex]\scriptsize \tau[/latex]) is a measure of the ability of a force to cause a rotation. Torque is calculated as the product of the force and its perpendicular distance from the pivot: [latex]\scriptsize \tau =F{{r}_{\bot }}[/latex].
• The unit of torque is newton metres ([latex]\scriptsize \displaystyle \text{N}\text{.m}[/latex]).
• The two conditions for equilibrium are:
  1. [latex]\scriptsize \displaystyle \Sigma {{F}_{x}}=0[/latex] and [latex]\scriptsize \displaystyle \Sigma {{F}_{y}}=0[/latex]
  2. [latex]\scriptsize \displaystyle \Sigma {{\tau }_{{\text{clockwise}}}}=\Sigma {{\tau }_{{\text{anticlockwise}}}}[/latex]

Unit 1: Assessment

Suggested time to complete: 15 minutes

1. Define torque.
2. Explain why it is easier to push open a door when you apply a force further from the hinge than close to the hinge.
3. Two children push on opposite sides of a door during play. Both push horizontally and perpendicular to the door. One child pushes with a force of [latex]\scriptsize 17.5\text{ N}[/latex] at a distance of [latex]\scriptsize 0.6\text{ m}[/latex] from the hinges, and the second child pushes at a distance of [latex]\scriptsize 0.45\text{ m}[/latex]. What force must the second child exert to keep the door from moving? Assume friction is negligible.

The full solutions are at the end of the unit.

Unit 1: Solutions

Exercise 1.1

1. .
   [latex]\scriptsize \begin{align*}\tau &=F{{r}_{\bot }}\\&=(55)(0.85)\\&=46.75\text{ N}\text{.m}\end{align*}[/latex]
2. .
   [latex]\scriptsize \begin{align*}\tau &=F{{r}_{\bot }}\\&=(165)(0.14)\\&=23.1\text{ N}\text{.m}\end{align*}[/latex]
3. .
   [latex]\scriptsize \begin{align*}\Sigma \tau &=0\\\therefore {{\tau }_{{\text{anticlockwise}}}}&={{\tau }_{{\text{clockwise}}}}\\(27\times 9.8)(1.2)&=(m\times 9.8)(0.8)\\317.52&=7.84m\\\therefore m&=40.5\text{ kg}\end{align*}[/latex]

Back to Exercise 1.1

Unit 1: Assessment

1. Torque is a measure of the ability of a force to cause a rotation.
2. The hinge serves as the pivot about which the rotation occurs. Torque is the product of the force and the perpendicular distance to where the torque is applied. Because the relationship is directly proportional, it means that a longer distance will multiply the force. Pushing against the door further away from hinge therefore allows you to exert a smaller force to obtain the same effect.
3. .
   [latex]\scriptsize \begin{align*}\Sigma \tau &=0\\\therefore {{\tau }_{{\text{anticlockwise}}}}&={{\tau }_{{\text{clockwise}}}}\\(17.5)(0.6)&={{F}_{2}}(0.45)\\\therefore {{F}_{2}}&=\displaystyle \frac{{10.5}}{{0.45}}=\\&=23.3\text{ N}\end{align*}[/latex]

Back to Unit 1: Assessment