Mechanical Properties of matter: Describe, analyse and apply principles heat and thermal properties of solids and liquid
Unit 2: Latent heat
Emma Harrage
Unit outcomes
By the end of this unit you will be able to:
- Define and calculate latent heat.
- Describe and differentiate between vapour pressure and boiling point.
- Describe evaporation as a cooling process and factors that affect it.
What you should know
Before you start this unit, make sure you can:
- Understand the expansion of a liquid. Refer to level 3 subject outcome 5.5 unit 1 to revise this.
Introduction
is defined as the heat or energy that is absorbed or released during a phase change of a substance. It could either be from a gas to a liquid or liquid to solid or vice versa. Latent heat is related to a heat property called .
Latent heat
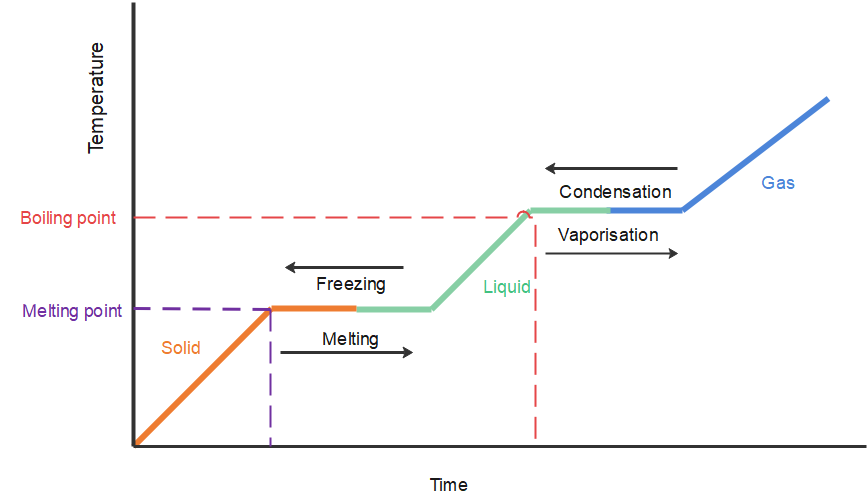
When ice (a solid) melts, it turns into water (a liquid); this is called fusion. When water (a liquid) boils, it turns into steam (a gas); this is called vaporisation.
When a substance changes from one state to another, energy is either absorbed or liberated. This heat energy is called the latent heat, and part of it is the energy used to overcome the forces of attraction between the molecules.
It is important to realise that no temperature change occurs during the change of state. The temperature will only rise or fall when the entire specimen has changed from one state to the other. As far as the mechanism is concerned, latent heat is the work that is needed to overcome the attractive forces that hold molecules and atoms together in a substance. However, an important point that we should consider regarding latent heat is that the temperature of the substance remains constant.
Let us look at an example. Suppose a solid substance is changing to a liquid, it needs to absorb energy to push the molecules into a wider, more fluid volume. Similarly, when a substance changes from a gas phase to a liquid, their density levels also need to go from a lower to a higher level and so the substance then needs to release or lose energy so that the molecules come closer together. This energy change that is required by a substance to either freeze, melt or boil is said to be latent heat.
It is clearly useful to know the energy required to change the state of unit mass of the substance. This is known as the specific latent heat and is defined as follows: The specific latent heat is the energy required to change the state of [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of the substance.
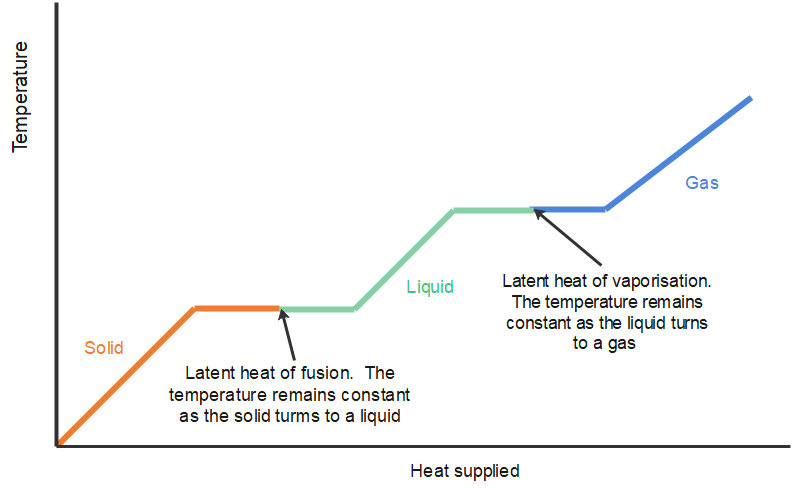
Latent heat is measured in units of joules per kilogram ([latex]\scriptsize \displaystyle \text{J/kg}[/latex]). The energy is referred to as latent, or hidden, because in phase changes, energy enters or leaves a system without causing a temperature change in the system; so, in effect, the energy is hidden.
If we look at how much energy is needed to melt a kilogram of ice at [latex]\scriptsize \displaystyle 0~^\circ\text{C}[/latex] to produce a kilogram of water at [latex]\scriptsize \displaystyle 0~^\circ\text{C}[/latex], we find that [latex]\scriptsize \displaystyle \text{334 kJ}[/latex] of energy is needed. So, [latex]\scriptsize \displaystyle 334\text{ kJ}[/latex] is the energy needed to melt a kilogram of ice. This is a large amount of energy as it represents the same amount of energy needed to raise the temperature of [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of liquid water from [latex]\scriptsize \displaystyle 0~^\circ\text{C}~\text{to}~79~^\circ\text{C}[/latex]. Even more energy is required to vaporise water; it would take [latex]\scriptsize \displaystyle 2256\text{ kJ}[/latex] to change [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of liquid water at the normal boiling point of [latex]\scriptsize 100 ~^\circ\text{C}[/latex] to steam (water vapour).
This example shows that the energy for a phase change is enormous compared to energy associated with temperature changes without a phase change.
Phase changes can have a tremendous stabilising effect even on temperatures that are not near the melting and boiling points, because evaporation and condensation (the conversion of a gas into a liquid state) occur even at temperatures below the boiling point.
For example, the fact that air temperatures in humid climates rarely go above [latex]\scriptsize \displaystyle 35.0~^\circ\text{C}[/latex], is because most heat transfer goes into evaporating water into the air. Similarly, temperatures in humid weather rarely fall below the because enormous amounts of heat is released when water vapour condenses.
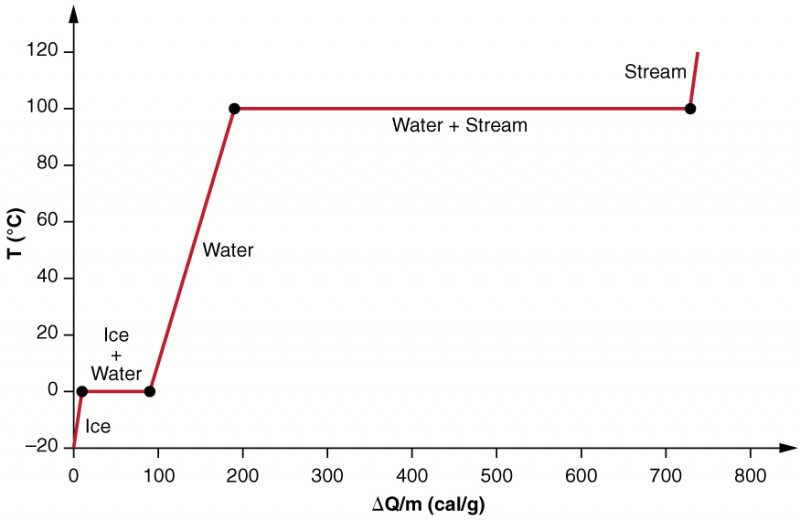
In figure 3, you can see that the system is constructed so that no vapour evaporates while ice warms to become liquid water, and so that, when vaporisation occurs, the vapour remains in the system. The long stretches of constant temperature values at [latex]\scriptsize \displaystyle 0~^\circ\text{C}[/latex] and [latex]\scriptsize \displaystyle 100~^\circ\text{C}[/latex] reflect the large latent heat of melting and vaporisation, respectively.
The effects of phase change are examined more precisely, by adding heat to a sample of ice at [latex]\scriptsize -20~^\circ\text{C}[/latex] (as shown in figure 3). The temperature of the ice rises , absorbing heat at a constant rate of [latex]\scriptsize 0.50~\text{cal/g.}^\circ\text{C}[/latex] until it reaches [latex]\scriptsize 0~^\circ\text{C}[/latex]. Once at this temperature, the ice begins to melt until all the ice has melted, absorbing [latex]\scriptsize \displaystyle \text{79}\text{.8 cal/g}[/latex] of heat. The temperature remains constant at [latex]\scriptsize 0~^\circ\text{C}[/latex] during this phase change. Once all the ice has melted, the temperature of the liquid water rises, absorbing heat at a new constant rate of [latex]\scriptsize 1.00~\text{cal/g.}^\circ\text{C}[/latex]. At [latex]\scriptsize 100~^\circ\text{C}[/latex], the water begins to boil, and the temperature again remains constant while the water absorbs [latex]\scriptsize \displaystyle \text{539 cal/g}[/latex] of heat during this phase change. When all the liquid has become steam vapour, the temperature rises again, absorbing heat at a rate of [latex]\scriptsize 0.482~\text{cal/g.}^\circ\text{C}[/latex].
Any material has two specific latent heats; the specific latent heat of fusion and the specific latent heat of vaporisation.
The is the thermal energy required to convert [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of solid to liquid with no change in temperature. This term is used when melting a solid or freezing a liquid.
- When a solid substance is melted, its temperature stays constant until all the substance has melted.
- The latent heat of fusion is the energy needed to break the forces of attraction between the molecules.
- If the substance in its liquid state is cooled, it will solidify at the same temperature as its melting point.
- When this happens, the particles are attracted together together into a rigid structure.
- Latent heat is transferred to the surroundings as the substance solidifies and the particles form stronger attractive forces.
The is the thermal energy required to convert [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of liquid to gas with no change in temperature. This term is used when vaporising a liquid or condensing a gas.
- When a liquid substance is heated, at its boiling point, the substance boils and turns into vapour.
- The latent heat of vaporisation is the energy needed by the particles to break away from their neighbouring particles in the liquid.
- If the substance in its gas state is cooled, it will condense at the same temperature as its boiling point.
- Therefore, latent heat is transferred to the surroundings as the substance condenses into a liquid and its particles form new intermolecular forces.
Calculating specific latent heat
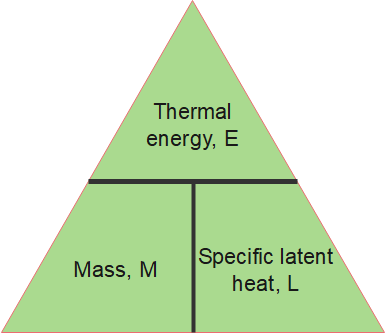
The amount of energy [latex]\scriptsize E[/latex] required to melt or vaporise a mass of [latex]\scriptsize m[/latex] with latent heat [latex]\scriptsize \displaystyle L[/latex] is:
[latex]\scriptsize \displaystyle E\text{ }=\text{ }mL[/latex]
Where:
[latex]\scriptsize E[/latex] = thermal energy required for a change in state, in joules ([latex]\scriptsize \displaystyle J[/latex])
[latex]\scriptsize m[/latex] = mass, in kilograms [latex]\scriptsize (\text{kg})[/latex]
[latex]\scriptsize \displaystyle L[/latex] = specific latent heat, in joules per kilogram ([latex]\scriptsize \displaystyle \text{J/kg}[/latex])
For context, the values of latent heat for water are:
Specific latent heat of fusion = [latex]\scriptsize \displaystyle 330\text{ kJ/kg}[/latex]
Specific latent heat of vaporisation = [latex]\scriptsize \displaystyle 2.26\text{ MJ/kg}[/latex]
Therefore, evaporating [latex]\scriptsize \displaystyle 1\text{ kg}[/latex] of water requires seven times more energy than melting the same amount of ice to form water.
Example 2.1
Calculate the energy transferred to the surroundings as [latex]\scriptsize \displaystyle 0.60\text{ kg}[/latex] of stearic acid changed state from liquid to solid. The specific latent heat of fusion of stearic acid is [latex]\scriptsize \displaystyle 199\text{ }000\text{ J/kg}[/latex].
Solution
Step 1: List the known quantities
Mass = [latex]\scriptsize \displaystyle m=0.60\text{ kg}[/latex]
Specific latent heat of fusion, [latex]\scriptsize \displaystyle L[/latex] = [latex]\scriptsize \displaystyle 199\text{ }000\text{ J/kg}[/latex]
Step 2: Write down the relevant equation
[latex]\scriptsize \displaystyle E\text{ }=\text{ }mL[/latex]
Step 3: Substitute in the values and calculate
[latex]\scriptsize \displaystyle E\text{ = 0}\text{.60 }\!\!\times\!\!\text{ 199 000 = 119 400 J}[/latex]
Exercise 2.1
- What is the name of the variable that tells you how much heat energy is required to change the state of a substance?
- Specific latent heat
- Specific heat capacity
- Capacity of materials to withstand heat
- What happens to the temperature of an object when it changes from a solid to a liquid?
- It increases
- It decreases
- It remains the same
- What happens to the temperature of an object when it changes from a liquid to a gas?
- It remains the same
- It decreases
- It increases
- Why does the temperature of ice remain at [latex]\scriptsize 0\text{ }^\circ \text{C}[/latex] when it is melting?
- Heat energy is moving the particles more quickly
- Heat energy is making the particles expand
- Heat energy is being used to overcome the forces between the particles
- .
- The melting point and boiling point of lead are [latex]\scriptsize \displaystyle 327\text{ }^\circ \text{C}[/latex] and [latex]\scriptsize \displaystyle 1774\text{ }^\circ \text{C}[/latex] respectively, and [latex]\scriptsize \displaystyle -219\text{ }^\circ \text{C}[/latex] and [latex]\scriptsize \displaystyle -183\text{ }^\circ \text{C}[/latex] for oxygen respectively.
- At [latex]\scriptsize \displaystyle -\text{ }200\text{ }^\circ \text{C}[/latex] will the oxygen be a solid, liquid, or gas?
- At [latex]\scriptsize \displaystyle 450\text{ }^\circ \text{C}[/latex] will the lead be a solid, liquid, or gas?
- The graph below shows how the temperature of a pure substance changes as it is heated.

- At what temperature does the substance melt?
- Identify the section of the graph with a letter X where the substance is a liquid only and with a letter Y where it exists as both a liquid and solid at the same time.
- If the heat applied to the substance provides [latex]\scriptsize \displaystyle 100\text{ J}[/latex] of heat energy every second, use the graph to calculate the energy required to melt this substance.
- If [latex]\scriptsize \displaystyle 1.5\text{ kg}[/latex] of the substance was used, use your answer to part (ii) to calculate the specific latent heat of fusion for this substance.
- The melting point and boiling point of lead are [latex]\scriptsize \displaystyle 327\text{ }^\circ \text{C}[/latex] and [latex]\scriptsize \displaystyle 1774\text{ }^\circ \text{C}[/latex] respectively, and [latex]\scriptsize \displaystyle -219\text{ }^\circ \text{C}[/latex] and [latex]\scriptsize \displaystyle -183\text{ }^\circ \text{C}[/latex] for oxygen respectively.
The full solutions are at the end of the unit.
Vapour pressure and boiling point
Vaporisation and boiling are two terms used to express the phase change of a liquid or a solid. Vaporisation is the phase change of a liquid or solid into its vapour. Boiling causes the phase change of a liquid into its vapour. Vaporisation gives rise to the of a closed system. The is the temperature at which a liquid is vapourised. Although these two terms are related to each other, there are differences between them. The main difference between vapour pressure and boiling point is that vapour pressure is a measurement of pressure whereas boiling point is a measurement of temperature.
What is vapour pressure?
Vapour pressure can be defined as the force exerted by the vapour.
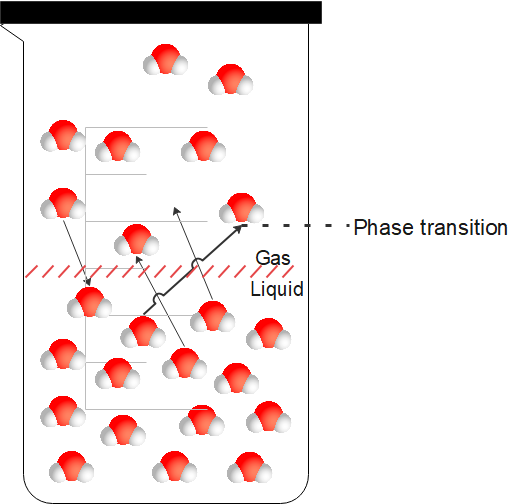
The vapour should meet the following conditions to exert the vapour pressure:
- The vapour should be in equilibrium with its liquid or solid phase.
- Vapour should be at a constant temperature.
- Both vapour and its condensed form should exist in a closed system.
Vapour pressure is related to the desire of molecules to escape the liquid or solid. Substances with high vapour pressure at normal temperature are . If the temperature remains constant, the vapour pressure also remains constant. But once the temperature increases, the average kinetic energy of liquid molecules increases, releasing more and more molecules from the liquid. As a result, the transition of liquid molecules into vapour is increased. So, the vapour pressure is also increased. At a certain temperature, the vapour pressure becomes equal to the external pressure exerted on the liquid or solid. The temperature at which this happens is called the boiling point of the liquid.
What is boiling point?
The boiling point is the temperature at which a liquid boils. In other words, it is the temperature at which the vapour pressure of a liquid is equal to the external pressure which is applied on the liquid by the surrounding environment.
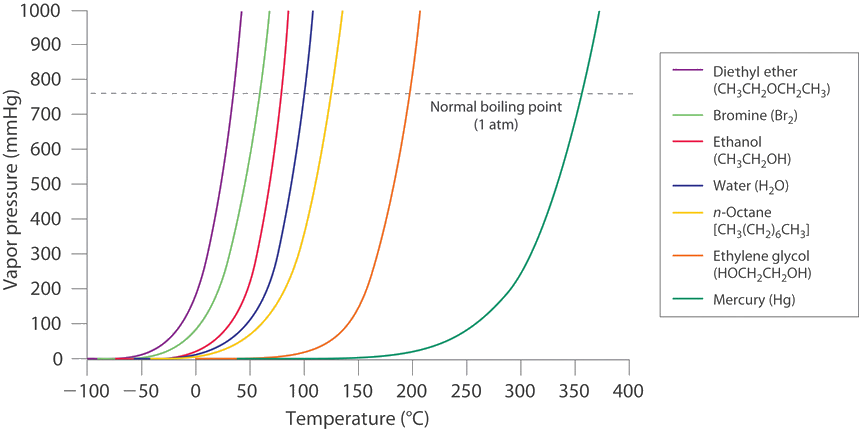
The boiling point of a liquid varies with the atmospheric pressure. Hence the value of the boiling point for a certain liquid is not always constant. The atmospheric pressures varies according to elevation. For example, water normally boils at [latex]\scriptsize \displaystyle 100\text{ }^\circ \text{C}[/latex] when the atmospheric pressure is [latex]\scriptsize \displaystyle 1[/latex] atm. But at higher altitudes, water boils at lower temperatures. That is because the vapour pressure should be equal to the atmospheric pressure for a liquid to boil. As the pressure at higher altitudes is lower, a low heat energy (temperature) is enough to meet the above criteria.
Even at temperatures below the boiling point, liquid molecules will become vapour due to a process called . Evaporation is the escaping of liquid molecules which are located on the surface of a liquid. These molecules are only loosely bound to the other molecules in the liquid; thus, they can easily detach from other molecules and escape the liquid as vapour. But in boiling, molecules located anywhere in the liquid can escape the liquid.

Vapour pressure and boiling point are inversely proportional. If the vapour pressure of a liquid is low, the boiling point is high, and vice versa. Vapour pressure is inversely proportional to the strength of the intermolecular forces of attraction.
So, weak intermolecular forces mean the liquid has a higher vapour pressure. Less heat energy needs to be applied to overcome the forces of attraction., and the boiling point will be quite low.
Conversely, if the intermolecular forces are strong, the molecules will be strongly attracted to each other. Fewer molecules break away and convert into vapours. Hence, the vapour pressure will be low. In this case, the boiling point will be high
| Vapour pressure | Boiling point |
| The force exerted by vapour released from a liquid in a closed space or container. | The temperature at which the vapour pressure is equal to the external pressure applied on the liquid. |
| Defined for a closed system with a constant temperature. | Defined for a system with a constant pressure. |
| Related to both a liquid and solid phase. | Related to a liquid phase only. |
Exercise 2.2
- The lower the boiling point, the ___________ the intermolecular forces.
- stronger
- weaker
- When the atmospheric pressure equals the equilibrium vapour pressure _________ occurs.
- freezing
- melting
- boiling
- sublimation
- The atmospheric pressure of four cities is listed below:
City Atmospheric pressure (kPa) i Johannesburg [latex]\scriptsize 1~035[/latex] ii London [latex]\scriptsize \displaystyle 1~013[/latex] iii Tokyo [latex]\scriptsize \displaystyle 1~090[/latex] iv Jericho [latex]\scriptsize \displaystyle 1~009[/latex] - Based on the above data, in which city will water boil first?
- List the cities in order from first to last.
The full solutions are at the end of the unit.
Evaporation
Evaporation can generally be defined as a process in which a liquid or solid is transformed into vapour.
When you spray perfume on your body, your body feels slightly cooler. The same effect happens with acetone and water. This is an effect of evaporation or the change of matter from its liquid state to its vapour state. The only difference is the rate at which the coolness is felt. In the case of acetone, the part of your body that is in contact with the liquid will cool the fastest. This happens because the evaporation rate of acetone is higher than that of water or perfume.
Evaporation is a form of vaporisation that usually happens on the surface of liquids, and it involves the transition of the liquid particles into the gaseous phase. Therefore, this process is said to involve a change in the state of matter of liquids. The surrounding gas must not be saturated with the substance which is evaporating. The liquid particles will escape and enter the surrounding air as a gas when a molecule near the surface gains enough energy to overcome the vapour pressure. The energy extracted from the liquid as evaporation occurs will decrease the temperature of the liquid, resulting in a process known as evaporative cooling.
Evaporation causes cooling naturally. To change its state, the matter must either gain or lose energy. In the case of change of phase from liquid to gas, molecules of matter require kinetic energy. So, the liquid takes this energy from its surroundings. When energy transfer occurs, it results in an increase or decrease in temperature of the substance, depending on whether the energy is being transferred from the substance to the surroundings or vice versa.
Although there is an increase in temperature of the substance until its boiling point is reached, there is no observable heat transfer in the phase change. The molecules of the substance absorb heat energy continuously from the surroundings and cool the surroundings until they reach the boiling point, after which they start to break free from the liquid and turn into vapour. Since there is no change in temperature until the evaporation process is complete i.e. the entire liquid changes into vapour, the amount of energy required for this phase change is called the latent heat of vaporisation. This heat will not change the temperature reading on a thermometer.
Applications of evaporative cooling
Our bodies use this process to cool down by sweating. . Water from our body evaporates, taking energy from our body in the process and this results in the lowering of our body temperature.
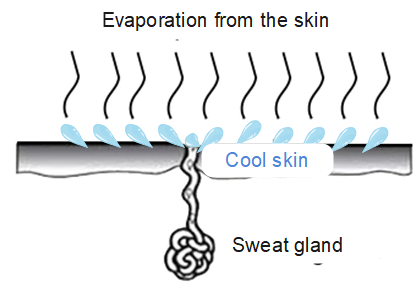
An air-cooling system also utilises this principle, and so is more effective on hot, dry days. The basic principle behind the working of an air cooler is evaporative cooling. On a hot, dry day, the temperature is high, and humidity is low, so the evaporation rate is high. The water takes energy from the air and is converted to vapour. This makes the air cooler.

Exercise 2.3
- Which process causes cooling naturally?
- Esterification
- Eutrophication
- Evaporation
- Equilibrium
- Which of the following is an example of evaporation?
- Melting of butter
- Drying of clothes
- Conversion of water to ice
- Conversion of ice to water
- If someone gets wet in chilly weather, why will they feel colder?
- The water is colder than the air.
- The water will evaporate from their skin and take heat from their body to do so.
- Water is a good conductor.
- Water is a good insulator.
- Explain how evaporation causes cooling.
The full solutions are at the end of the unit.
Summary
In this unit you have learnt the following:
- To change a solid into a liquid, or a liquid into a gas, requires heat energy. This heat energy allows the change of state to happen, and the temperature remains constant during the process.
- The amount of energy required to change the state of a substance depends upon the mass and characteristics of that substance.
- When a substance changes from one state to another, the temperature remains constant. For example, when heat energy is added to ice at its melting point ([latex]\scriptsize 0\text{ }^\circ \text{C}[/latex]), it changes into the same mass of water at [latex]\scriptsize 0\text{ }^\circ \text{C}[/latex].
- The energy required to change the state of a material is known as the latent heat.
- The amount of energy required to change the state of that material is known as the specific latent heat of that substance.
- A solid substance at its melting point has less energy than the same mass of the substance when it is a liquid at the same temperature.
- Energy is required to change the phase of a substance, such as the energy to overcome intermolecular forces in a block of ice so it may melt.
- The amount of energy required to change the state of [latex]\scriptsize 1\text{ kg}[/latex] of that material is known as the specific latent heat of that substance. It can be:
- specific latent heat of fusion (solid to liquid) or
- specific latent heat of vaporisation (liquid to gas).
- During a phase change energy may be added or removed from a system, but the temperature will not change. The temperature will change only when the phase change is completed.
- Vaporisation is the phase change of a liquid or solid into its vapour.
- Boiling causes the phase change of a liquid into its vapour.
- Evaporation is a phase change from a liquid to a gas and causes natural cooling.
Unit 2: Assessment
Suggested time to complete: 15 minutes
- Copy the following sentences into your notebook and fill in the blanks:
- A solid must be given __________ _________ heat of _________ before it can be melted into a liquid.
- A liquid must be given ___________ ____________ heat of _________ before it can be boiled into a gas.
- The energy needed to melt a solid is used to overcome the ___________ ________ between the molecules so that they can move __________ freely.
- The specific latent heat of fusion is the amount of energy (measured in _________) needed to melt _________ kg of a solid into a ___________ without changing its ___________.
- Explain why a burn from steam at [latex]\scriptsize 100\text{ }^\circ \text{C}[/latex] is so much worse than a burn from water at [latex]\scriptsize 100\text{ }^\circ \text{C}[/latex].
- An electric kettle produces [latex]\scriptsize \displaystyle 2000\text{ J}[/latex] of energy each second. It is filled with water, weighed, and switched on. After coming to the boil, it is left on for a further [latex]\scriptsize \displaystyle 120\text{ seconds}[/latex] and is then switched off. It is found to be [latex]\scriptsize \displaystyle 90\text{ g}[/latex] lighter. Calculate the specific latent heat of vaporisation from this data.
- .
- Explain how perspiration helps to keep you cool.
- When walking on a mountain in wet weather a person’s clothing became soaked. Explain why this could be dangerous to the person’s health.
The full solutions are at the end of the unit.
Unit 2: Solutions
Exercise 2.1
- a
- c
- a
- c
- .
- .
- liquid
- liquid
- .
- [latex]\scriptsize \displaystyle 110\text{ }^\circ \text{C}[/latex]
- X should be between [latex]\scriptsize \displaystyle 110[/latex] and [latex]\scriptsize \displaystyle 440~^\circ\text{C}[/latex] (on the line between [latex]\scriptsize \displaystyle 3[/latex] and [latex]\scriptsize \displaystyle 5.8[/latex] seconds)
Y should be on the flat line at [latex]\scriptsize \displaystyle 110~^\circ\text{C}[/latex] (between [latex]\scriptsize \displaystyle 0.6[/latex] and [latex]\scriptsize \displaystyle 2.8[/latex] seconds) - it melts for [latex]\scriptsize \displaystyle 2.2[/latex] seconds; energy is [latex]\scriptsize \displaystyle 100\text{ J}[/latex] every second, so:
[latex]\scriptsize \displaystyle 100\text{ }x\text{ }2.2\text{ }=\text{ }220\text{ J}[/latex] of energy is needed to melt the substance - [latex]\scriptsize \displaystyle L=\displaystyle \frac{E}{m}=\displaystyle \frac{{220}}{{1.5}}=\text{ }146.7\text{ J/Kg}[/latex]
- .
Exercise 2.2
- b
- c
- .
- iv) Jericho
- iv, ii, i, iii
Exercise 2.3
- c
- b
- b
- Evaporation causes cooling because the process requires heat energy. The energy is taken in by the molecules when they convert from liquid into gas, and this causes cooling on the original surface.
Unit 2: Assessment
- .
- specific latent, fusion
- specific latent, vaporisation
- intermolecular forces/attractive forces, more
- [latex]\scriptsize \displaystyle \text{J/kg}[/latex], [latex]\scriptsize 1[/latex], liquid, temperature
- When [latex]\scriptsize 100\text{ }^\circ \text{C}[/latex] water touches your hand it cools and releases energy, this energy can be calculated using the specific heat capacity. When [latex]\scriptsize 100\text{ }^\circ \text{C}[/latex] steam touches your hand, the steam condenses and changes into a liquid; it releases the latent heat of vaporisation. It then cools releasing the same energy as the water. This means that the steam releases more energy.
- Specific latent heat = energy/mass [latex]\scriptsize \displaystyle L=\displaystyle \frac{E}{m}=\text{ }2000\text{ x }\displaystyle \frac{{120}}{{0.09}}=\text{ }\displaystyle \frac{{240,000}}{{0.09}}=\text{ }2\text{ }670\text{ }000\text{ J/Kg}[/latex]
- .
- perspiration evaporates, takes in (latent) heat from skin and the skin cools
- water evaporating from damp clothing needs heat from body, causing the person to get very cold
Media Attributions
- Fig 1 © DHET is licensed under a CC BY (Attribution) license
- Fig 2 © DHET is licensed under a CC BY (Attribution) license
- Fig 3 © Lumen is licensed under a CC0 (Creative Commons Zero) license
- Fig 4 © DHET is licensed under a CC BY (Attribution) license
- Fig 5 © olivia_calloway is licensed under a CC BY-SA (Attribution ShareAlike) license
- Fig 6 © DHET is licensed under a CC BY (Attribution) license
- Fig 7 © Libretext is licensed under a CC BY-NC-SA (Attribution NonCommercial ShareAlike) license
- Fig 8 © DHET is licensed under a CC BY (Attribution) license
- Fig 9 © DHET is licensed under a CC BY (Attribution) license
- Fig 10 © DHET is licensed under a CC BY (Attribution) license
the heat required to convert a solid into a liquid or vapour, or a liquid into a vapour, without change of temperature
a measure of the total energy of a chemical system for a given pressure, and is given the symbol H
the atmospheric temperature (varying according to pressure and humidity) below which water vapour begins to condense and dew can form
in a straight or nearly straight line
the amount of energy that must be supplied to a solid substance (typically in the form of heat) to trigger a change in its physical state and convert it into a liquid
the amount of heat added to or removed from a substance to produce a change in phase, to vaporise a substance
the force exerted by the vapour released by a liquid or solid substance in a closed container or space
the temperature at which the vapour pressure is equal to the external pressure applied on the liquid
likely to change suddenly and unexpectedly, especially by getting worse
the process of turning from liquid into vapour
